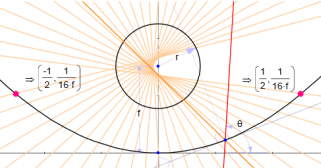
Student Projects
We present the reports from a number of student projects.
These summer research projects were completed by year 11 students working at Saltire Software as part of the Saturday Academy's Apprenticeship in Science and Engineering program.